Explanation
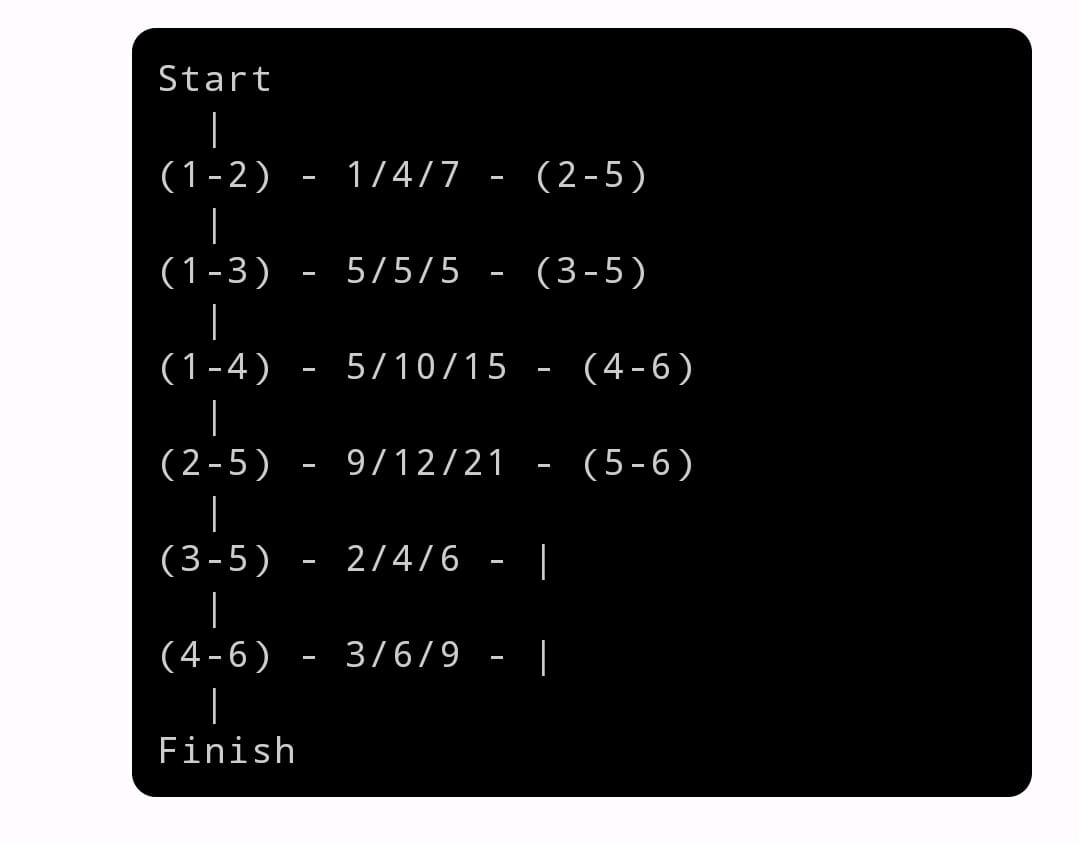
(i) To draw the network diagram and identify the critical path, we can use the given information about activities and their optimistic, most likely, and pessimistic times.
The critical path is the longest path in the network, which is determined by adding the expected times for each activity. The expected time is calculated using the formula: Expected Time = (Optimistic + 4 * Most Likely + Pessimistic) / 6.
- Activity (1-2): Expected Time = (1 + 4 * 5 + 7) / 6 = 5
- Activity (1-3): Expected Time = (5 + 4 * 5 + 5) / 6 = 5
- Activity (1-4): Expected Time = (5 + 4 * 10 + 15) / 6 = 10
- Activity (2-5): Expected Time = (9 + 4 * 12 + 21) / 6 = 13.5
- Activity (3-5): Expected Time = (2 + 4 * 4 + 6) / 6 = 4
- Activity (4-6): Expected Time = (3 + 4 * 6 + 9) / 6 = 6
The critical path is the longest path, which is (1-4) – (2-5) – (5-6) with a total expected time of 10 + 13.5 + 6 = 29.5 days.
(ii) To calculate the probability of project completion within 19 days, we can use the Z-score and the Central Limit Theorem. We need to find the Z-score for 19 days, given the mean completion time and standard deviation. The mean completion time is the sum of the expected times on the critical path, which is 29.5 days. The standard deviation can be calculated using the formula: Standard Deviation = (Pessimistic – Optimistic) / 6.
Standard Deviation = (15 – 1) / 6 = 2.33 (approximately)
Z-score
Z = (19 – 29.5) / 2.33 = -4.5 / 2.33 ≈ -1.93
Using a Z-table or calculator, you can find the probability of completion within 19 days for a Z-score of -1.93. This probability will be very close to 0 because 19 days is significantly below the mean completion time.
(iii) Again use the Z-score and the Central Limit Theorem.
First, calculate the Z-score for 21 days:
Z = (21 – 29.5) / 2.33 = -8.5 / 2.33 ≈ -3.65
Now, using a Z-table or calculator, find the probability of completion beyond 21 days for a Z-score of -3.65. This probability will be very close to 0.
Therefore, the probability that the company will have to pay a penalty of Rs. 16,000 is very low, nearly zero, based on the given completion time estimates.

